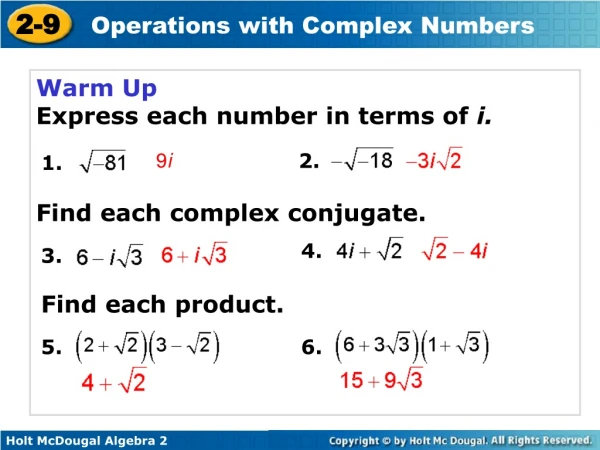
Complex numbers - PowerPoint PPT Presentation
View Complex numbers PowerPoint (PPT) presentations online in SlideServe. SlideServe has a very huge collection of Complex numbers PowerPoint presentations. You can view or download Complex numbers presentations for your school assignment or business presentation. Browse for the presentations on every topic that you want.