00:00

Understanding Vector Cross Product in Physics

The vector cross product yields a new vector perpendicular to the original two vectors, with a magnitude that can be zero or positive depending on the angle between the vectors. This concept is crucial in physics for determining forces on moving charges in magnetic fields and requires understanding unit vectors, the right-hand rule, and the determinant of a 3x3 matrix.

machero
Download Presentation

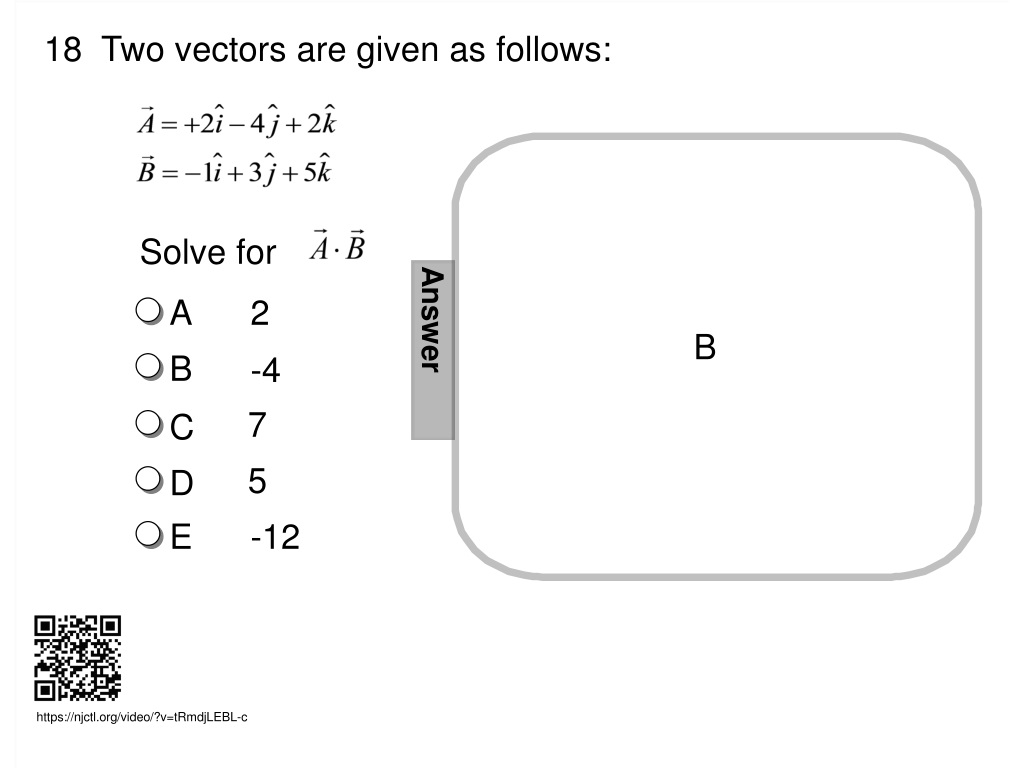
Understanding Vector Cross Product in Physics

An Image/Link below is provided (as is) to download presentation Download Policy: Content on the Website is provided to you AS IS for your information and personal use and may not be sold / licensed / shared on other websites without getting consent from its author. Content is provided to you AS IS for your information and personal use only. Download presentation by click this link. While downloading, if for some reason you are not able to download a presentation, the publisher may have deleted the file from their server. During download, if you can't get a presentation, the file might be deleted by the publisher.

E N D

Presentation Transcript

  1. 18 Two vectors are given as follows: Solve for Answer A B 2 -4 7 5 -12 B C D E https://njctl.org/video/?v=tRmdjLEBL-c

  2. 19 Solve for the angle between vectors and Answer 98o 278o 57o 85o 124o A B C D E A https://njctl.org/video/?v=ak5u5eVQHzs

  3. 20 Two vectors are given: The angle between vectors A and B, in degrees, is: Answer o A B C D E 117 o 76 150 o o 29 161 B o https://njctl.org/video/?v=BkKKnh__U9c

  4. Vector Cross Product The vector cross product yields a vector quantity and its magnitude is defined as: The cross product can be viewed as the "projection" of the magnitude of A on a line perpendicular to vector B (Asinθ), multiplied by the magnitude of B. The magnitude of this product can be positive or zero depending on angle θ. θ ranges from 0 to 1800. The vector cross product is a minimum when the two vectors are in line, θ = 00. It is a maximum when θ = 900. https://njctl.org/video/?v=N1WKTxZt_xE

  5. Vector Cross Product The vector cross product yields a vector quantity and its magnitude is defined as: Since the cross product results in a vector, it must also have a direction. In previous physics courses, the concept of the "right hand rule" was developed to give this direction. Physicists don't use the right hand rule to solve complex problems - they use the vector cross product.

  6. Vector Cross Product The vector cross product yields a vector perpendicular to the plane ab described by the two vectors a and b. The vector cross product is not a commutative operation as shown on the figure: By User:Acdx - Self- made, based on Image:Crossproduct.png, Public Domain, https://commons.wikimedi a.org/w/index.php?curid= 4436304 The magnitude of the cross product of a and b is:

  7. Vector Cross Product Why does the vector cross product yield a new vector perpendicular to the plane described by the original two vectors? Examine the unit vectors. Each pair of unit vectors (that are not parallel to each other - their cross product is zero) yields the third unit vector. Use the right hand rule to verify this! More complex vectors behave the same way. When the order of the unit vectors is reversed, you get the opposite direction for the cross product.

  8. Vector Cross Product The Magnetism unit of this course showed that the magnitude of the force on a moving charge in a magnetic field is: It is a maximum when v and B are perpendicular to each other. This is now written more powerfully and elegantly as: This notation gives both the direction and the magnitude. The direction of the resultant vector, F is perpendicular to the plane described by the vectors v and B. The magnitude is:

  9. Vector Cross Product Apply the definition of the vector cross product to the unit vectors: To remember the cross product rules for the unit vectors, write out the unit vectors in order (twice): Going from left to right, you get a positive result: Going from right to left, you get a negative value:

  10. Vector Cross Product Using the properties of the unit vectors, we find the vector in component notation. By User:Acdx - Self- made, based on Image:Crossproduct.png, Public Domain, https://commons.wikimedi a.org/w/index.php?curid= 4436304

  11. Vector Cross Product Nobody can be expected to memorize this expression: There is another method, using linear algebra and taking the determinant of a 3 x 3 matrix (the pair of vertical lines on either side of the components of each vector). Don't worry if you don't know the math - the next slide shows how to work the equation below.

  12. Vector Cross Product 1. 2. 3. 3. 1.Create the 3 x 3 matrix using the unit vectors and the vector components and put the vertical lines on either side, representing the need to take its determinant. 2.The 3 x 3 determinant breaks into three 2 x 2 determinants. To find the component, draw an imaginary vertical line down from and eliminate those vector components from the determinant. 3.Repeat for and , placing a negative sign in front of .

  13. Vector Cross Product 1. 2. 3. 3. 4. 5. 4. Cross multiply within each 2 x 2 matrix, starting from the top left corner, putting a negative sign in front of the second product. 5. Reverse the order of the component to make all the components positive (only to match what was shown earlier).

  14. 16 In the figure, find the magnitude of the vector cross product of vectors A and B . 12 30 25 20 10 A B C D E Answer 4 C 7 65o 45o 6 https://njctl.org/video/?v=0TvRmA6a29I

  15. 17 Two vectors are given as follows: Solve for Answer A B C D E D See next slide for worked out solution. https://njctl.org/video/?v=DmHBFu0YwRU

  16. Two vectors are given as follows: Worked Out Solution Solve for

  17. 21 Two vectors are given: Solve for the magnitude of Answer 33 29 25 21 17 A B C D E A See next slide for worked out solution. https://njctl.org/video/?v=-oJOuHBjJTs

  18. Two vectors are given: Worked Out Solution Solve for the magnitude of

More Related